Get Your Adverbs Here!

OTHER RESOURCES:

Quest for Adverbs Game
Adverbs of Manner Games
List Of 100+ Common Adverbs

Divide & Check!

November STEM Challenge

In November, we are going to design free-standing pendulums that can draw or paint as they swing to and fro!

A pendulum is an object, hung from a fixed point, that swings freely back and forth under the action of gravity. The back and forth movements of a pendulum are called oscillations.

(I could watch that sand pendulum allll daaaay 😍)

Kids love to ride the swings at the playground. The motion of a tire swing demonstrates the physics of a pendulum. The swing is supported by chains that are attached to a fixed point at the top of the swing set, which allow it to move freely back and forth. 

The Foucault Pendulum is named for the French physicist Jean Foucault, who used it to demonstrate the rotation of the earth in 1851. It was the first experiment to give simple, direct evidence of the Earth's rotation.

Check out this cool video that explains how the Foucault Pendulum proves the rotation of our amazing planet, Earth. 

Let's design a pendulum!

Research pendulums and use items from around your house to complete this challenge.

Send Mrs. Sol pictures or movies of your experiment to share in class.

DUE NOVEMBER 15

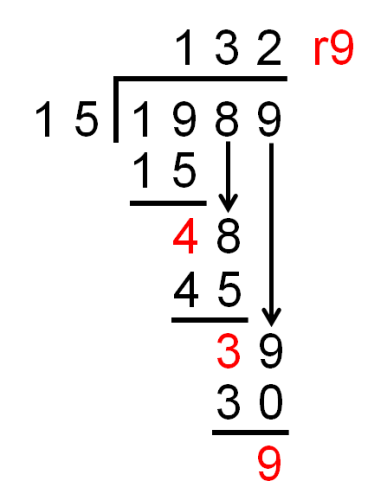
Divide and Conquer!

The standard algorithm for division may work for you...

DIVIDE

MULTIPLY

SUBTRACT

BRING DOWN

CHECK!

BUT, I have two new strategies that give you a little more freedom with numbers!

You can choose numbers without having to be so precise with each step of the process. 

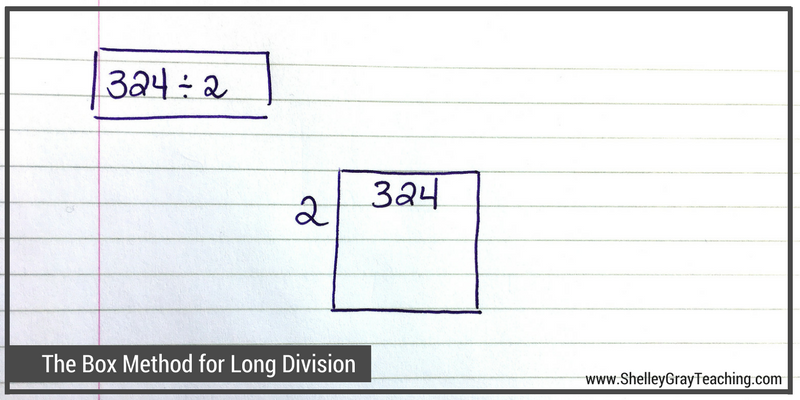
Suppose that we want to solve the equation 

324 ÷ 2

First, draw a box with the dividend on the inside and the divisor on the outside.

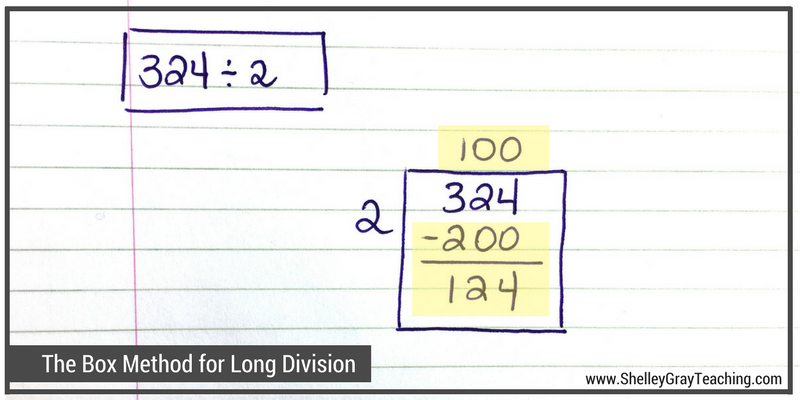
Then, think in multiples to find a number that is close, but does not go over.
Think 10s, 100s, 1000s!

2 x 100 = 200

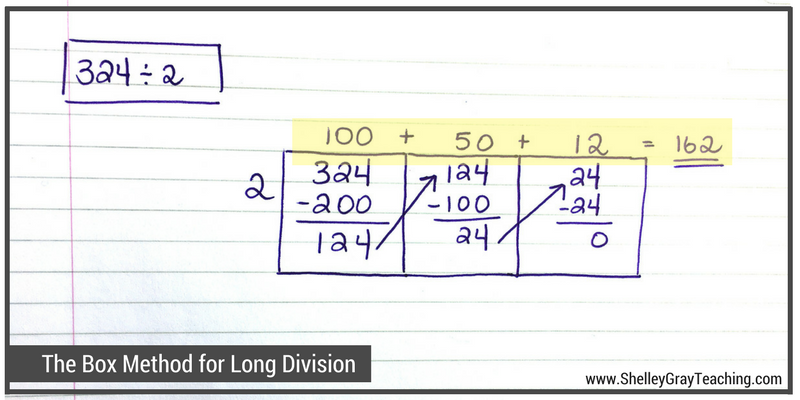
Subtract and write the answer in the next box.

Repeat until you are left with a number that is smaller than the divisor.

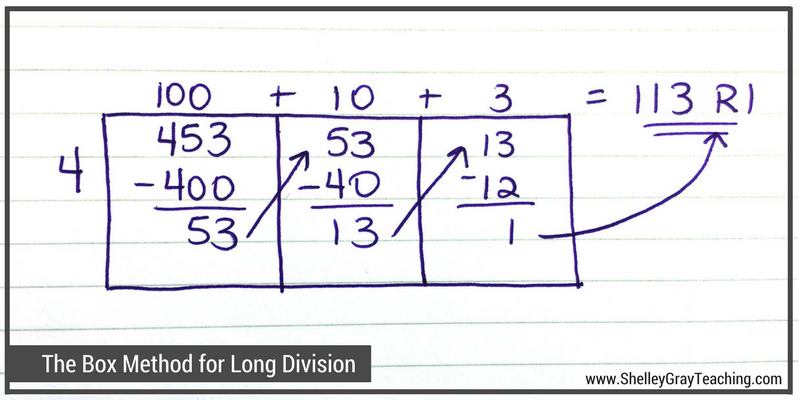
Let’s take a look at one more example. 

In this example, we will solve 

453 ÷ 4

Draw a box with the dividend on the inside and the divisor on the outside.

Think in multiples to find a number that is close, but does not go over.

Think: 10s, 100s, 1000s!

Think 2s and 5s!

Subtract and write the answer in the next box.

Keep going until you are left with a number that is smaller than the divisor.

When all is said and done, there is a 1 left over...

that's your REMAINDER! 

113 r 1    or     113 1/4

The area model strategy works with 2-digit divisors too! 

Here's an example from page 20 in your Math Activity Book:

Step 1: Think of a few easy X facts for the divisor.

Step 2: Subtract from the dividend an easy multiple of the divisor (e.g. 100x, 10x, 5x, 2x). Record the partial quotient in a column to the right of the problem.

Step 3: Repeat until the dividend has been reduced to zero or the remainder is less than the divisor.

Step 4: Add the partial quotients to find the quotient.

Let’s solve

679 ÷ 5

Click on the images above for a closer look.

Visit Shelly Gray's math website for the videos above and many other helpful math lessons! 

What's the Point of View?

This week we are learning about the narrator's

POINT OF VIEW in a story!