The standard algorithm for division may work for you...
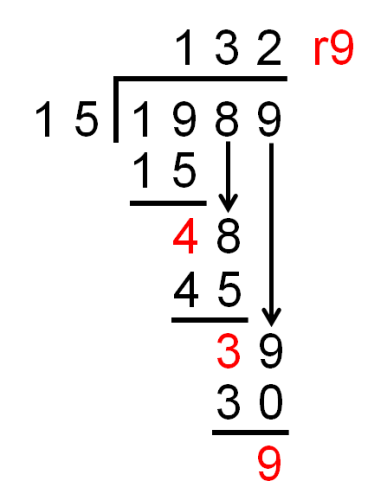
DIVIDE
MULTIPLY
SUBTRACT
BRING DOWN
CHECK!
BUT, I have two new strategies that give you a little more freedom with numbers!
You can choose numbers without having to be so precise with each step of the process.
Suppose that we want to solve the equation
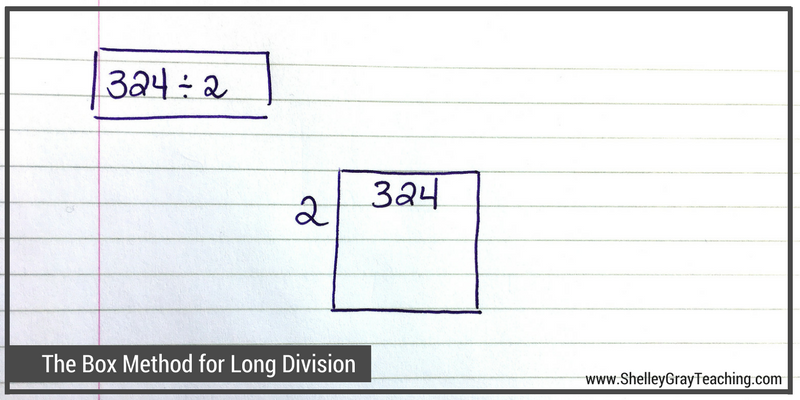
324 ÷ 2
First, draw a box with the dividend on the inside and the divisor on the outside.
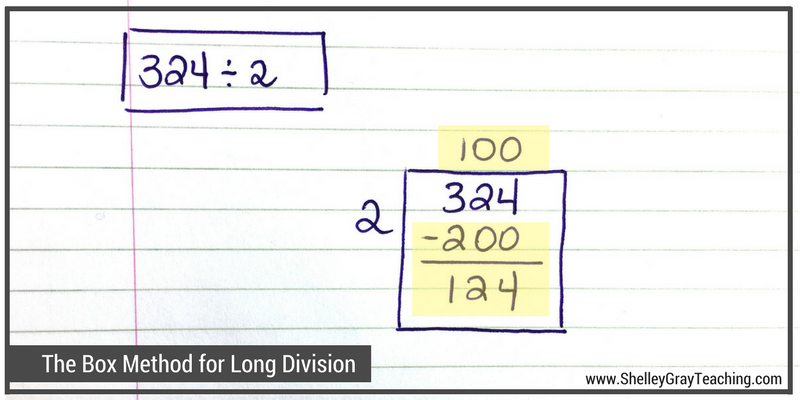
Then, think in multiples to find a number that is close, but does not go over.
Think 10s, 100s, 1000s!
Think 10s, 100s, 1000s!
2 x 100 = 200
Subtract and write the answer in the next box.
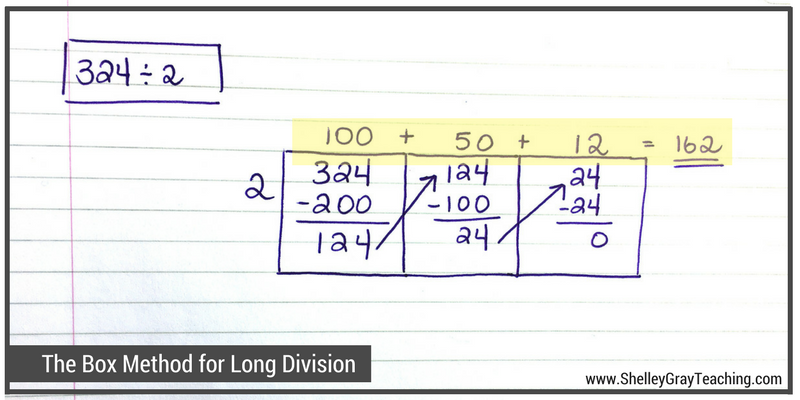
Repeat until you are left with a number that is smaller than the divisor.
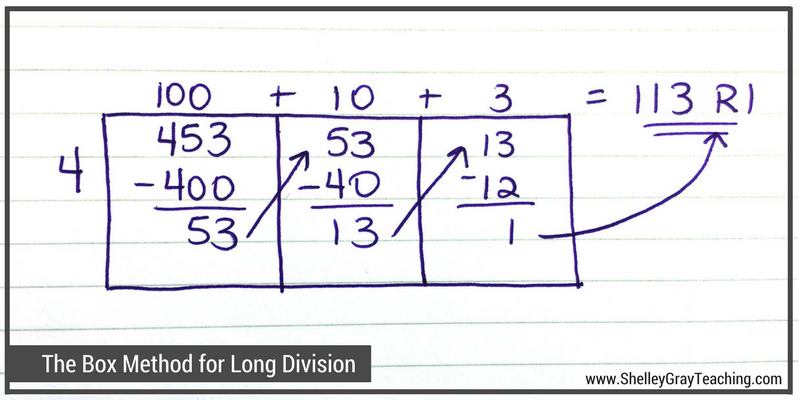
Let’s take a look at one more example.
In this example, we will solve
453 ÷ 4
Draw a box with the dividend on the inside and the divisor on the outside.
Think in multiples to find a number that is close, but does not go over.
Think: 10s, 100s, 1000s!
Think 2s and 5s!
Subtract and write the answer in the next box.
Keep going until you are left with a number that is smaller than the divisor.
When all is said and done, there is a 1 left over...
that's your REMAINDER!
113 r 1 or 113 1/4
The area model strategy works with 2-digit divisors too!
Here's an example from page 20 in your Math Activity Book:
Step 1: Think of a few easy X facts for the divisor.
Step 2: Subtract from the dividend an easy multiple of the
divisor (e.g. 100x, 10x, 5x, 2x). Record the partial quotient in a column to
the right of the problem.
Step 3: Repeat until the dividend has been reduced to zero
or the remainder is less than the divisor.
Step 4: Add the partial quotients to find the quotient.
Let’s solve
679 ÷ 5
679 ÷ 5
Click on the images above for a closer look.
Visit Shelly Gray's math website for the videos above and many other helpful math lessons!